SBI PO Quantitative Aptitude Questions and Answers PDF Download (Prelims): Candidates can check the details of SBI PO Quantitative Aptitude Prelims Questions With Answers from this article. Applicants who have started preparing for the SBI PO Exam need to focus on this article for the Quantitative Aptitude Questions For SBI PO. From the below sections, we have given the complete information regarding the SBI PO Quantitative Aptitude Online Exam Questions and Answers.
★★ SBI PO Prelims Mock Tests ★★
SBI PO Quantitative Aptitude Questions and Answers
To make it easy for the aspirants, we have arranged a direct link to download the SBI PO Quantitative Aptitude Questions with Answers Pdf at the end of this article. So, start preparing for the test using the given Quantitative Aptitude Questions & Answers For SBI PO .
Quantitative Aptitude Questions For SBI PO – Prelims
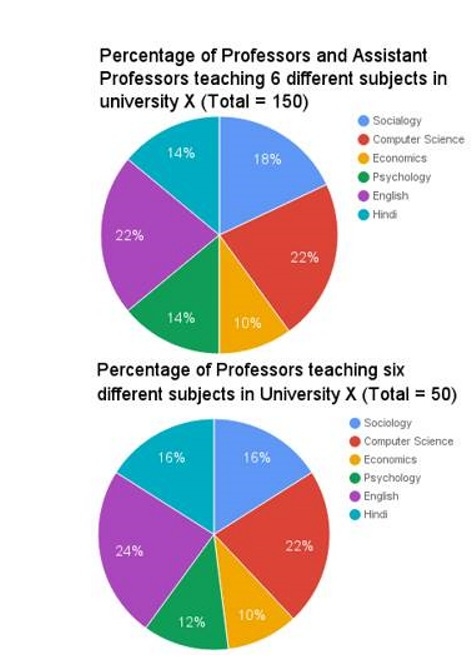
(1-5) Direction: Study the following pie charts below and answer the questions that follow:
1. What is the central angle corresponding to the total number of professors and assistant professors teaching Hindi?
46.8 °
50.4 °
43.2 °
39.6 °
45.2 °
Solution:
from I chart,
Angle = (14/100) * 360 = 50.4 °
2. What percent of professors are teaching Psychology and Sociology together out of the total number of professors and assistant professors teaching these two subjects together?
175/6
97/4
155/6
87/4
133/6
Solution:
Total professors teaching Psychology and Sociology together = ((16 + 12)/100) * 50 = 14
Total number of professors and assistant professors teaching Psychology and Sociology together = ((18 + 14)/100) * 150 = 48
Required % = 14/48 * 100 = 175/6 %
3. The Total number of assistant professors teaching Economics and English together is what percent more than the total number of professors teaching these two subjects together?
88
55
98
92
82
Solution:
Total number of professors teaching Economics and English together = (((10+24)/100) * 50 = 17
Total number of professors and assistant professors teaching Economics and English together = ((10+22)/1000 * 150 = 48
Total number of assistant professors teaching Economics and English together = 48 – 17 = 31
Required % = (31 – 17)/17 * 100 = 82%
4. What is the approximate average number of professors teaching computer science, Economics, English, and sociology?
9
8
10
11
None of these
Solution:
Professor teaching –
Computer Science = 22/100 * 50 = 11
Economics = 10/100 * 50 = 5
English = 24/100 * 50 = 12
Sociology = 16/100 * 50 = 8
Average = (11 + 5 + 12 + 8)/4 = 9
5. Assistant Professor who teaching Psychology is how much percent of Professor, who teach English?
150%
140%
120%
125%
None of these
Solution:
Total Professor & Assistant Professor teaching psychology= 150×14%= 21
Professor teaching Psychology= 12% of 50= 6
Assistant professor teaching Psychology= 21-6= 15
Now Professor teaching English= 24% of 50= 12
so required % of Assistant professor= 15 × 100/12=125%
6. A started a project by investing Rs. X. After 6 months, B invested Rs. 4,000 more than what A had invested. At the end of the year, the ratio of overall profit to that of B’s was 7 : 3. What is the value X?
2000
5000
8000
4000
6000
Solution:
The ratio of the equivalent capitals of A and B for 1 month:
X × 12 : (X + 4000) × 6
2X : X + 4000
Sum of their ratios:
2X + X + 4000 = 3X + 4000
The value of X:
X+4000/3X+4000 = 3/7
7X + 28000 = 9X + 12000
2X = 16000
X = 8000
7. The average weight of a group of 20 students increases by 1.5kg when one teacher joins the group. The average weight of the group was 28 kg before the teacher joined the group. What is the weight of the teacher?
58kg
59.5kg
57.5kg
55kg
None of these
Solution:
A simple approach to solving this type of question rather than a mathematical approach is the observational approach.
Let us see how
When the teacher joined the group the average weight is increased by 1.5kg and the total no. of people in a group also increases to 21 from 20.
So, the total increase in weight of the group =
Previous Avg. Weight + (Increase in Avg. Wight)×(Total number of people in the group after the teacher joins)
= 28 + 1.5×21 kg = 28+31.5 kg = 59.5 kg
Hence teacher’s weight = 59.5 kg
8. Three years ago, the ratio of the age of a son and his father was 1:9. The present ratio of age of the son and his mother is 4:21. If 6 years ago, his father was 6 years older than his mother, what is the present age of his mother?
42 years
45 years
39 years
48 years
None of these
Solution:
ATQ,
(S-3)/(F-3) =1/9 ⇒ 9S – F = 24..(i)
& S/M = 4/21 & F-M = 6
S/(F-6) =4/21 ⇒ 21S – 4F = -24…(ii)
Solving i & ii
We get,
S= 8 ⇒ M = 42 yrs
9. Rahul has a total sum of Rs.6150, he invests some part of this amount in scheme A at a rate of 15% p.a. for 2 years and rests part in scheme B at a rate of 30% p.a. for 2 years. Simple interest earned from both schemes is equal. Find how much amount he invested in scheme B.
Rs. 2000
Rs. 2050
Rs.2100
Rs. 2150
Rs.2200
Solution:
Let Rahul invests Rs. P in schemes A and Rs. (6150 – P) on scheme B
A.T.Q,
The interest earned from both the scheme is equal.
P*15*2 / 100 = (6150-P)*30*2 / 100
P = 2(6150 – P)
P + 2P = 12300
3 P = 12300
P = 4100
Therefore, the amount invested in scheme B = Rs. (6150 – 4100) = Rs. 2050
10. (A + C) can complete a piece of work in 4 days and (B + C) can complete the work in 8 days. A alone can complete the work in 6 days. Find the time that B will require to complete the work alone?
24 days
12 days
6 days
8 days
none of these
Solution:
Let the total work = T
(A + C) can complete a piece of work in 4 days.
So, 1 day work of (A + C) = T / 4
A alone can complete the work in 6 days.
So, 1 day work of A = T / 6
Now, 1 day work of C = T / 4 – T / 6 = T / 12
(B + C) can complete the work in 8 days.
So, 1 day work of (B + C) = T / 8
1 day work of B = T / 8 – T / 12
Time taken by B to complete the work alone = T / T / 24 = T × 24 / T
= 24 days
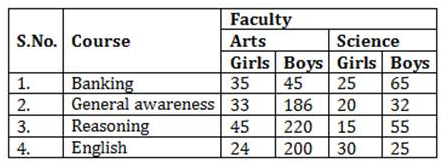
(11-15) Directions: Read the following table carefully and answer the question given below.
Distribution of students according to professional courses:
11. If 60% of boys and 70% of girls are successful in the courses taken by them what is the combined pass percentage? (approx.)
54%
58%
62%
76%
none of these
Solution:
Total number of boys = 45+186+220+200+65+32+55+25= 828
∴Number of successful boys = 60% of 828 = 496.8
∴Total number of girls=35+33+45+24+25+20+15+30 = 227
∴Number of successful girls = 70% of 227 = 158.9
∴Combined pass percentage = ((496.8+158.9)×100)/((828+227) )%
= (655.70*100)/1055% = 62.15% = 62%
12. In which professional course has the maximum number of students for both arts and science.
Banking
General Awareness
Reasoning
English
none of these
Solution:
Banking = (35+45+25+65) = 170
General awareness = (33+186+20+32) = 271
Reasoning = (45+220+15+55) = 335
English = (24+200+30+25) = 279
So maximum number of students in Reasoning.
13. By approximately what percent the number of students doing Banking and Reasoning is more than the number of students doing English only?
83%
81%
85%
88%
92%
Solution:
Number of students in Banking = (35+45+25+65) = 170
Number of students in Reasoning = (45+220+15+55) = 335
And number of students in English only = (24+200+30+25) = 279
∴ Required percentage = ((170+335-279))/279×100%
= 81%
14. Students of art faculty doing only General Awareness is what percent of the total number of students of art faculty?
24%
36%
32%
28%
none of these
Solution:
Required percentage
= [Students doing GA from Art/ Total Art faculty]*100%
= ((33 + 186)) / 788 × 100%
= 27.79% =28%
15. What is the ratio of the total number of girls in banking and reasoning to the total number of boys in banking and reasoning. (Approximately).
24:77
26:79
22:81
28:83
none of these
Solution:
Total no of girls in banking and reasoning = (35+25+45+15) = 120
Total no of boys in banking and reasoning = (45+65+220+55) = 385
Required Ratio: 120 : 385 = 24: 77
16. A dishonest seller sold his goods at a cost price but he uses a weight of 900g instead of 1kg, then finds the profit percentage earned by the seller.
11(1/9)
4(5/8)
10(2/7)
2(7/5)
6(8/17)
Solution:
Let the cost of 1 kg be Rs 100
Cost of 900g= Rs 90
Profit= 100-90= Rs 10
Profit percentage = 10*100/90= 11(1/9) %
17. The variety of wheat sold by a grocer contained 10% low-quality wheat. What quantity of good quality wheat should be added to 250 kg of this variety of wheat so that the percentage of low-quality wheat becomes 5%?
285 kg
250 kg
135 kg
150 kg
None of these
Solution:
Here, 10% of 250 kg = 25 kg
So, Low-quality wheat = 25 kg
Suppose x units of good quality wheat are added to the mixture.
Then, ATQ
[25/(250+x)] = 5/100
x = 250 kg
To become the 5% low quality of wheat, we add 250 kg of more wheat.
18. A person takes 13 hours to travel a distance of 87 km. He covers some part of his journey by Bicycle and some part by Bus. The speed of the Bicycle is 3 km/h and the speed of Bus is 11 km/h. Then find the distance covered by the Person on the Bicycle?
21 km
66 km
87 km
13 km
none of the these
Solution:
Total distance = 87 km
Total time is taken = 13 hours
Let the distance covered by the Person on Bicycle = x km
So, the distance covered by the Person by Bus = 87 – x
Speed of Bicycle = 3 km/h
Speed of Bus = 11 km/h
according to the question :
x / 3 + (87-x) / 11 = 13
Multiply both side by 33(LCM of 3 & 11) to eliminate fraction :
11x + 3(87 – x) = 429
11x + 261 – 3x = 429
8x = 168
x = 21 km
19. Puja, Ram, and Taimoor invested Rs. 5000, Rs. 10000 and Rs. 5000 respectively in a company. After some time Puja withdrawn her investment from the company and Ram increased his investment by some amount at the same time. If at the end of six-year the profit shared was in ratio 5:38:15, then what is the increased amount of Ram investment?
2000
3000
4000
5000
6000
Solution:
NOTE: If A invests Rs x for p duration and B invests Rs y for q duration, then (A’s share of profit): (B’s share of profit)=xp:yq
Let t be the time after which Puja withdrew her investment and x be the final amount after t years, which is invested for 6-t years.
In six years-
(Money invested × Duration of investment) for puja = 5000 × t
(Money invested × Duration of investment) for Ram = 10000 × t + x(6-t)
(Money invested × Duration of investment) for Taimoor = 5000 × 6 = 30000
At the end of six year the profit shared was in ratio 5:38:15
5000t : 10000 × 6 + x(6 – t) : 30000 = 5 : 38 : 15
⇒ 5000t / 30000 ⇒ 5 / 15 t = 2 years
Putting t = 2 in
⇒ 60000 + 4x / 30000 = 38 / 15
⇒ 60000 + 4x = 76000
⇒ x = Rs. 4000
20. A student’s marks in 5 subjects in class 12th are in the ratio 5:4:7:8:6. The maximum marks in each subject are the same. If he scored 54% in the examination, find the number of subjects in which he scored more than 60%.
2
1
3
4
None of these
Solution:
Let the maximum marks in each subject be 100.
Let his marks in 5 subjects be 5a, 4a, 7a, 8a, and 6a respectively.
He scored 54% in this examination.
Thus, 5a + 4a + 7a + 8a + 6a = 54% of 500
⇒ 30a = 270
⇒ a = 9
Marks in subjects are 45, 36, 63, 72 and 54
The number of subjects in which he scored more than 60 is 2.
(21 – 25) Direction: Read the paragraph below and answer the questions that follow:
In the Delhi Auto Expo 2016, 4.8 lakh people visited in three days. 42% of these people belonged to Delhi. 67% were Indians and the rest were equally divided between Germany, Japan, and South Korea. To help the visitors, 1200 students from Delhi University and 600 students from Ambedkar University volunteered. Out of the 1200 DU volunteers, only 50 could communicate with the Germans, 100 could communicate to Japanese, and 50 to Koreans. Out of the 600 Ambedkar University volunteers, only 50 could communicate with the Germans, 50 could communicate to Japanese, and 50 to Koreans.
21. What is the average number of German visitors handled by both University volunteers if volunteer work is equally divided among all the volunteers?
1056
538
518
548
528
Solution:
Number of visitors = 480000
Number of German visitors = 11% of 480000
= 0.11 * 480000 = 52800
Number of Delhi University volunteers that are fluent in German = 50
Number of Ambedkar University volunteers that are fluent in German = 50
total volunteer by both universities who handled german= 50+50=100
The number of visitors handled by each volunteer = 52800/100 = 528
22. What is the average number of visitors handled by a volunteer?
247
257
267
277
287
Solution:
Total number of visitors = 4.8 lakh
Total number of volunteers = 1200 + 600
Average visitors handled by a volunteer = 480000/1800 = 4800/18 = 267
23. What is the difference between visitors from Delhi and visitors from Japan?
147800
146800
148800
148600
147200
Solution:
Total number of visitors = 4.8 lakhs
Visitors from Delhi = 42% of 4.8 lakhs = 2.016 lakhs = 201600
Visitors from Japan = (100 – 67)/3 = 33/3 = 11% of 4.8 lakhs = 52800
Difference between visitors from Delhi and Japan = 201600 – 52800 = 148800
24. What is the total number of Korean visitors handled by Ambedkar university volunteers if volunteer work is equally divided among all the volunteers?
24400
25400
26400
27400
None of these
Solution:
Number of visitors = 480000
Number of Korean visitors = 11% of 480000
= 0.11 * 480000 = 52800
Number of Ambedkar University volunteers that are fluent in Korean = 50
Total number of volunteers fluent in Korean = 50 + 50 = 100
Since the Ambedkar and Delhi university volunteers are equal in number, Ambedkar university volunteers will handle 50% of the Korean visitors i.e. 26400.
25. What is the approx. daily average of Japanese visitors handled by Delhi university volunteers if volunteer work is equally divided among all the volunteers?
15967
11733
15777
15767
15987
Solution:
Number of visitors = 480000
Number of Japanese visitors = 11% of 480000
= 0.11 * 480000 = 52800
Number of Ambedkar University volunteers that are fluent in Japanese = 50
Number of Delhi University volunteers that are fluent in Japanese = 100
Total Number of volunteers fluent in Japanese = 150
Since the volunteers are divided in the ratio of 2:1, the work will be divided between Delhi University and Ambedkar University students in the same ratio
Therefore the number of visitors by Delhi University volunteers per day = (2/ 3 * 52800 )/ (total no of days) = 35200/3 = 11733
26. Direction: What will come in place of the question mark (?) in the following number series?
0, 5, 18, 43, 84, 145, ?
220
240
260
280
230
Solution:
0 5 18 43 84 145 ?
5 13 25 41 61 85
8 12 16 20 24
4 4 4 4
So, ? = 230
27. Direction: What will come in place of the question mark (?) in the following number series?
10, 17, 48, 165, 688, 3475, ?
27584
25670
21369
20892
20735
Solution:
10 × 1 + 7 = 17
17 × 2 + 14 = 48
48 × 3 + 21 = 165
165 × 4 + 28 = 688
688 × 5 + 35 = 3475
3475 × 6 + 42 = 20892
So, ? = 20892
28. Direction: What will come in place of the question mark (?) in the following number series?
12, 14 , 32, 102, 416, 2090, ?
15522
12552
13525
17552
12450
Solution:
12 × 1 + 2 = 14
14× 2 + 4 = 32
32 × 3 + 6 =102
102 × 4 + 8 = 416
416 × 5 + 10 = 2090
2090 × 6 + 12 = 12552
So, ? = 12552
29. Direction: What will come in place of the question mark (?) in the following number series?
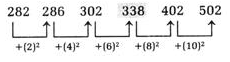
282, 286, 302, ?, 402, 502
366
318
326
338
None of these
Solution:
30. Direction: What will come in place of the question mark (?) in the following number series?
2.5, 4, ?, 10, 14.5, 20, 26.5
8
7.5
6
5.5
6.5
Solution:
The pattern of the number series is:
2.5 + 1.5 =4
4 + 2.5 = 6.5
6.5 + 3.5 = 10
10 + 4.5 =14.5
14.5 + 5.5 = 20
20 + 6.5 = 26.5
31. Direction: What will come in place of question mark(?) in the given number series?
3, 7, 13, 27, ?, 107, 213
41
47
53
54
None of these
Solution:
The pattern of the series is:
3 × 2 + 1 = 7
7 × 2 – 1 = 13
13 × 2 + 1 = 27
27 × 2 – 1 = 53
53 × 2 + 1 = 107
107 × 2 – 1 = 213
32. In a river, the ratio of the speed of current and speed of the boat in still water is 3:4 and the ratio of the speed of stream and speed of another boat in still water is 4:5. What is the ratio of the speeds of the first and second boat in still water?
14:9
16:15
5:6
5:7
9:10
Solution:
Let the Speed of the stream be 12 km/hr, then
For the first boat:
Speed of stream : speed of boat = 3 : 4 = 12 : 16
⇒ Speed of first boat = 16 km/hr
For the second boat:
Speed of stream : speed of boat = 4 : 5 = 12 : 15
⇒ Speed of second boat = 15 km/hr
Hence, the required ratio = 16:15.
33. 19 years ago, the average age of a woman and her daughter was 47.5 years. At present, two times the woman’s age is equal to five times the daughter’s age. 19 years hence, what will be the age of the daughter?
55 years
47 years
57 years
59 years
None of these
Solution:
Let the present age of woman be 5x years, then
The present age of daughter age = (2 × 5x)/5 = 2x years
According to question
(5x – 19) + (2x – 19) = 47.5 × 2
⇒ 7x – 38 = 95
⇒ x = (95 + 38)/7 = 19
19 years hence the age of daughter = 2x + 19 = 38 + 19 = 57 years
34. The marks scored by Riya Joshi in three subjects are in the ratio 4:5:6. Riya Joshi scored over an aggregate of 60% in the exam. If the maximum marks in each subject are the same, in how many subjects did Riya Joshi score more than 70% marks?
1
2
3
0
Can’t be determined
Solution:
Let the maximum marks in each subject be 100
So, Riya Joshi’s total score = 60% of (100+100+100) = 0.6(300) = 180
As per the question,
Let Riya Joshi have scored 4x,5x, and 6x in the three subjects.
Now, 4x + 5x + 6x = 180
15x = 180
x = 12
Therefore, marks in the three subjects are 48,60,72
Hence, he has scored more than 70 in exactly one subject.
Hence, option A is correct.
35. Tony marked all toy cars in his shop 40% higher. He gave a 35% discount on 60% of the total toy cars, and a 12% discount on 25% of the total toy cars. Find the discount percentage Tony should have given on the remaining toy cars to get an overall profit of 4.3% on the sale of all toy cars.
5%
15%
10%
20%
25%
Solution:
Let, Tony has 100 cars and the cost price of each car is Rs. 100
Then, the marked price of each car = 1.4 * 100 = 14
And, selling price of 100 cars = 100 * 100 * 104.3 / 100 = Rs. 10430
The selling price of 60 cars = 60 * 140 * 65 / 100 = Rs. 5460
The selling price of 25 cars = 25 * 140 * 88 / 100 = Rs. 3080
Let Tony gave x% discount on the remaining cars (100 – 65- 20 = 15 Cars)
According to the question,
15 * 140 * ((100 – x) / 100) + 5460 + 3080 = 10430
2100 * ((100 – x) / 100) = 1890
100 – x = 90
x = 10%
SBI PO Quantitative Aptitude Prelims Questions With Answers – Important Links
| SBI PO Quantitative Aptitude Questions & Answers | |
| To Download SBI PO Quantitative Aptitude Questions and Answers PDF – Prelims Exam |
Click Here |
For more details on the SBI PO Quantitative Aptitude Questions and Answers, applicants can visit our site which is Freshers Now.